Medicine and Allied Sciences
Engineering and Architecture
Management and Business Administration
Law
Animation and Design
Media, Mass Communication and Journalism
Finance & Accounts
Computer Application and IT
Pharmacy
Hospitality and Tourism
Competition
School
Study Abroad
Arts, Commerce & Sciences
Learn
Online Courses and Certifications

NEET PG 2024 Cut off Reduce by NBEMS - Qualifying Percentile & Branch-wise Expected Cutoff

Home
NEET
Study Material
Refraction And Dispersion Of Light Through A Prism MCQ - Practice Questions with Answers

Refraction And Dispersion Of Light Through A Prism MCQ - Practice Questions with Answers

Edited By admin | Updated on Sep 25, 2023 25:23 PM | #NEET

Syllabus
Application Process
Exam Pattern
Admit Card
Preparation Tips
Result
Cutoff
Counselling Process
Answer Key
Mock Test
Exam Analysis
Question Paper
College Predictor
Eligibility Criteria
Dates
College Predictor
Rank Predictor
Accepting Colleges
Study Material

Quick Facts

Refraction Through A Prism 1, Refraction Through A Prism 2, Dispersion Of Light 1 is considered one of the most asked concept.
51 Questions around this concept.

Solve by difficulty

The angle of incidence for a ray of light at a refracting surface of a prism is 45°. The angle of the prism is 60°. If the ray suffers minimum deviation through the prism, the angle of minimum deviation and refractive index of the material of the prism respectively, are:

A

45°;

Correct Answer

Wrong Answer

B

30°; $\sqrt{2}$

Correct Answer

Wrong Answer

C

45°; $\sqrt{2}$

Correct Answer

Wrong Answer

D

30°;

Correct Answer

Wrong Answer

The refracting angle of a prism is A, and refractive index of the material of the prism is cot(A/2). The angle of minimum deviation is:

A

90°-A

Correct Answer

Wrong Answer

B

180°+ 2A

Correct Answer

Wrong Answer

C

180°-3A

Correct Answer

Wrong Answer

D

180°-2A

Correct Answer

Wrong Answer

A ray of light is incident at an angle of incidence i, on one face of prism of angle A (assumed to be small) and emerges normally from the opposite face. If the refractive index of the prism is $\mu$ , the angle of incidence i is nearly equal to:

A

$\mu A$

Correct Answer

Wrong Answer

B

$\frac{\mu A}{2}$

Correct Answer

Wrong Answer

C

$\frac{A}{\mu}$

Correct Answer

Wrong Answer

D

$\frac{A}{2 \mu}$

Correct Answer

Wrong Answer

NEET 2024: Cutoff (OBC, SC, ST & General Category)

NEET 2024 Admission Guidance: Personalised | Study Abroad

NEET 2025: Syllabus | Most Scoring concepts | NEET PYQ's (2015-24)

NEET PYQ's & Solutions: Physics | Chemistry | Biology

The angle of a prism is 'A'. One of its reflecting surfaces is silvered. Light rays falling at an angle of incidence 2A on the first surface returns back through the same path after suffering reflection at the silvered surface. The refractive index $\mu$ , of the prism is:

A

$2\sin A$

Correct Answer

Wrong Answer

B

$2\cos A$

Correct Answer

Wrong Answer

C

$\frac{1}{2}\cos A$

Correct Answer

Wrong Answer

D

$\tan A$

Correct Answer

Wrong Answer

A beam of light consisting of red, green and blue colours is incident on a right angled prism. The refractive index of the material of the prism for the above red, green and blue wavelengths are 1.39, 1.44 and 1.47, respectively.

The prism will:

A

separate all the three colours from one another

Correct Answer

Wrong Answer

B

not separate the three colours at all

Correct Answer

Wrong Answer

C

separate the red colour part from the green and blue colours

Correct Answer

Wrong Answer

D

separate the blue colour part from the red and green colours

Correct Answer

Wrong Answer

A glass prism of refractive index 1.5 is immersed in water $(\mu=4 / 3)$ . A light beam incident normally on the face A B is totally reflected to reach the face B C if

A

$\mathrm{ \sin \theta>8 / 9 \\ }$

Correct Answer

Wrong Answer

B

$\mathrm{2 / 3<\sin \theta<8 / 9}$

Correct Answer

Wrong Answer

C

$\mathrm{ \sin \theta \leq 2 / 3 }$

Correct Answer

Wrong Answer

D

$\mathrm{\cos \theta \geq 8 / 9}$

Correct Answer

Wrong Answer

A luminous object is placed at a distance of 30cm from the convex lens of focal length 20cm. On the other side of the lens, at what distance from the lens, a convex mirror of radius of curvature 10cm be placed in order to have an upright image of the object coinciding with it

A

12 cm

Correct Answer

Wrong Answer

B

30 cm

Correct Answer

Wrong Answer

C

50 cm

Correct Answer

Wrong Answer

D

60 cm

Correct Answer

Wrong Answer

Pearson | PTE

Register now for PTE & Unlock 20% OFF : Use promo code: 'C360SPL20'. Valid till 31st DEC'24! Trusted by 3,500+ universities globally

In the figure shown a convex mirror of radius of curvature 20 cm is shown. An object O is placed in front of this mirror. Its ray diagram is shown. How many mistakes are there in the ray diagram (AB is its principal axis):

A

3

Correct Answer

Wrong Answer

B

2

Correct Answer

Wrong Answer

C

1

Correct Answer

Wrong Answer

D

0

Correct Answer

Wrong Answer

A ray of light is incident on the face of the equilateral triangle at an incident angle of 50^o. At this angle, minimum deviation occurs. This deviation is

A

30

Correct Answer

Wrong Answer

B

40

Correct Answer

Wrong Answer

C

50

Correct Answer

Wrong Answer

D

None of these

Correct Answer

Wrong Answer

Most Scoring concepts for NEET

This ebook serves as a valuable study guide for NEET exams, specifically designed to assist students in light of recent changes and the removal of certain topics from the NEET exam.

An equilateral prism has an angle of deviation $30^{\circ}$ when the angle of incidence is $60^{\circ}$ . The angle of deviation if a ray is incident normally on a surface is:

A

$50^{\circ}$

Correct Answer

Wrong Answer

B

$60^{\circ}$

Correct Answer

Wrong Answer

C

$90^{\circ}$

Correct Answer

Wrong Answer

D

$40^{\circ}$

Correct Answer

Wrong Answer

Concepts Covered - 4

Refraction Through A Prism 1

A prism is a transparent medium whose refracting surfaces are not parallel but are inclined to each other at an angle A which is also known as angle of the prism.

The angle of deviation $(\delta)$ -It is the angle between the emergent and the incident ray.

For the above figure $\begin{array}{l}{\delta=\left(i-r_{1}\right)+\left(e-r_{2}\right) \text { or } \delta=i+e-\left(r_{1}+r_{2}\right)} \end{array}$

and using $Using \ \ A=r_1+r_2 \ \ we \ \ get \ \ \delta =i+e-A$

Note-From the above formula, we can say that if we interchange i and e then also we will get the same value of $\delta$ .

The plot of $\delta \ \ vs \ \ i$

As shown in the above figure The graph is a parabola.

If we vary i between $0^0 \ \ to \ \ 90 ^0$

then $for \ \ 0 < i < e$ the value of $\delta$ decreases

and $for \ \ e < i < 90$ the value of $\delta$ increases

And when $i=e \ \ then \ \ \delta =\delta _{min}$

and when $i=90^0 \ \ or \ \ e=90^0 \ \ \ \ then \ \ \delta =\delta _{max}$

Grazing Incidence-When i = 90°, the incident ray grazes along the surface of the prism. This is known as grazing incidence.
Grazing Emergence- When e = 90°, the emergent ray grazes along the prism surface. This is known as grazing emergence.

This happens when the light ray strikes the second face of the prism at the critical angle for glass - air.

$I.e \ \ when \ \ \ \ r _2=\theta _c \ \ then \ \ \ e=90^0$

I.e For the prism of refractive index $\mu$ places in the air.

then $i=\sin ^{-1}[\sqrt{\mu ^{2}-1} \sin A-\cos A]$ then e = 90°

Refraction Through A Prism 2

Refractive index of prism $(\mu )$ in case of minimum deviation condition-

As we learned The angle of deviation $(\delta)$ for the prism is given as $\ \delta =i+e-A$

and from The plot of $\delta \ \ vs \ \ i$ we get $i=e \ \ then \ \ \delta =\delta _{min}$

$i.e \ \ \delta_{min} =i+e-A=i+i-A=2i-A\\\Rightarrow i=\frac{A+\delta _{min}}{2}$

For the prism of refractive index $\mu$ places in the air.

For the first surface, we can write $1*sini=\mu sinr_1$

similarly For the second surface, we can write $\mu sinr_2=1* sine$

using i=e we get $r_1=r_2$

$A=r_1+r_2=2r_1\\ \rightarrow r_1=\frac{A}{2}$

So $1*sini=\mu sinr_1$ will give us

$\Rightarrow 1*sin(\frac{A+\delta _{min}}{2})=\mu sin(\frac{A}{2})\\ \Rightarrow \mu =\frac{sin(\frac{A+\delta _{min}}{2})}{ sin(\frac{A}{2})}$

For thin films (i.e $A \ \ and \ \ \delta _{min} \ are \ small$ )

Then $sin(\frac{A+\delta _{min}}{2})=\frac{A+\delta _{min}}{2}$

and $sin(\frac{A}{2})=\frac{A }{2}$

So we get

$\mu = \frac{A+\delta _{min}}{A}\\ \Rightarrow \delta _{min}=A(\mu -1)$

condition of no emergence-

i.e A ray of light incidence on a prism of angle A & Refractive index $\mu$ will not emerge out of a prism

This will happen when $A> 2\theta _{c}$

where $\theta _{c}=$ critical angle

Dispersion Of Light 1

Dispersion of light -The splitting of white light into its constituent colors or wavelengths is called dispersion of light.

or

angular splitting of a ray of white light into a number of components and spreading in different directions is called diversion of light.

This phenomenon arises due to the fact that the refractive index varies with wavelength.

When white light is incident on the prism it will split itself into its constituent colors as shown in the below figure.

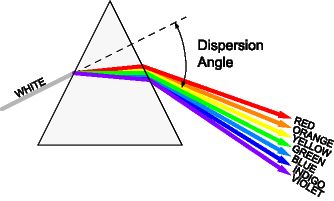
The deviation is given as $\delta =(\mu -1)A$

$Since \ \mu _{violet}>\mu _{red} \\ So \ \ \delta _{violet}>\delta _{red}$

Angular dispersion ( $\theta$ )- Angular separation between extreme colors

i.e $\boldsymbol{\theta}=\boldsymbol{\delta}_{V}-\boldsymbol{\delta}_{R}=\left(\boldsymbol{\mu}_{V}-\boldsymbol{\mu}_{R}\right) \boldsymbol{A} .$

It depends upon $\mu$ and A.

Dispersive power (ω)- Ratio of angular dispersion to mean deviation.

i.e $\omega=\frac{\delta_{v}-\delta_{r}}{\delta}$

where where $\delta$ is deviation of mean ray (especially yellow)

$\begin{aligned} using \ \ \delta _{v} &=\left(\mu_{v}-1\right) A, \delta _{r}=\left(\mu_{r}-1\right) A \\ we \ get \ \ \omega &=\frac{\mu_{v}-\mu_{r}}{\mu_{y}-1} \quad \text { where } \quad \mu_{y}=\frac{\mu_{v}-\mu_{r}}{2} \end{aligned}$

where

$\mu _{v}$ = Refractive index of violet

$\mu _{r}=$ Refractive index of red

$\mu _{y}=$ Refractive index of yellow

Dispersion Of Light 2

Condition for deviation without dispersion-

This means an achromatic combination of two prisms in which net(or) resultant dispersion is 0, but and deviation is produced as shown in the below figure.

$\begin{array}{ll}{\text { For the two prisms, }} \\ {\qquad \begin{aligned} \theta _{net}=0\Rightarrow \theta _1+\theta _2=0 \\ \left(\mu_{v}-\mu_{r}\right) A+\left(\mu'_{v}-\mu'_{r}\right) A '&=0 \\ \Rightarrow \quad A^{\prime}=\frac{\left(\mu_{v}-\mu_{r}\right) A}{\mu'_{v}-\mu'_{r}} & \end{aligned}}\end{array}$

where

$\mu _{v}$ = Refractive index of violet ( prism 1)

$\mu _{r}=$ Refractive index of red ( prism 1)

$\mu{}' _{v}$ = Refractive index of violet ( prism 2)

$\mu{}' _{r}=$ Refractive index of red ( prism 2)

Similarly

$\begin{array}{ll}{\text { For the two prisms, }} \\ {\qquad \begin{aligned} \theta _{net}=0\Rightarrow \theta _1+\theta _2=0 \\ & \end{aligned}}\end{array}$

$\omega \delta+\omega^{\prime} \delta^{\prime}=0\\ \Rightarrow \delta '=-\delta \frac{\omega }{\omega '}\\ \Rightarrow \delta_{net}=\delta +\delta '=\delta\left[1-\frac{\omega}{\omega^{\prime}}\right]$

where $\omega \ \ and \ \ \omega^{\prime}$ are the dispersive powers of the two prisms and their corresponding mean deviations are $\delta\ \ and \ \ \delta^{\prime}$ .

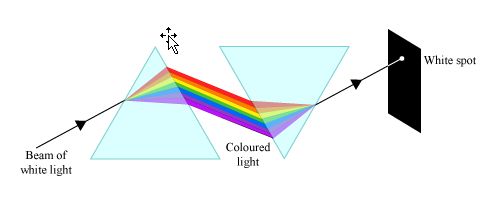
Condition for Dispersion without deviation-

A combination of two prisms in which deviation produced for the mean ray by the first prism is equal and opposite to that produced
by the second prism will give a dispersion of light without deviation.

This combination of two prisms is also called a direct vision prism.

i.e $\delta _{net}=0 \ \ while \ \ \theta _{net}\neq0$

As shown in the above figure as emergent rays from the second prism is parallel to the incident white ray of the prism 1.

this will give $\delta _{net}=0$ .

$\begin{aligned} \text { For zero deviation ,} \\ i.e \ \ \delta _{net}=0 \left( i.e \ \ \delta+\delta^{\prime}=0\right) \\ \Rightarrow \quad(\mu_y-1) A+\left(\mu_y^{\prime}-1\right) A'=0 \\ \Rightarrow \quad A^{\prime}=\frac{(\mu_y-1) A}{\left(\mu_y^{\prime}-1\right)} \end{aligned}$

and the Angular dispersion is given as

$\theta_{\text {net }}=\theta _1+\theta _2=\left(\omega \delta+\omega^{\prime} \delta^{\prime}\right)=\left(\omega \delta-\omega^{\prime} \delta\right)=\theta\left(1-\frac{\omega^{\prime}}{\omega}\right)$

Study it with Videos

Refraction Through A Prism 1

Refraction Through A Prism 2

Dispersion Of Light 1

Dispersion Of Light 2

Study other Related Concepts

Refraction And Dispersion Of Light Through A Prism Current Topic

Laws Of Reflection

Reflection On A Plane Mirror

Object And Image Velocity In Plane Mirror

Spherical Mirrors

Image Formation By Spherical Mirrors

Spherical Mirror Formula And Magnification

Refraction Of Light

Real Depth And Apparent Depth

Total Internal Reflection

Refraction Of Light Through Glass Slab

"Stay in the loop. Receive exam news, study resources, and expert advice!"

Get Answer to all your questions

NEET Articles

How Many Days Left for NEET 2025? - Countdown NEET 2025

Dec 19, 2024

NEET Biometric Attendance 2025 by NTA - Check Attendance Rules

Dec 19, 2024

NTA Report on NEET 2025 - Check Latest Update at exams.nta.ac.in

Dec 19, 2024

NEET OMR Sheet Sample 2025 PDF: Answer Sheet For Practice

Dec 19, 2024

Crack NEET 2025 in 5 Months: Preparation Strategy Tips & Study Plan

Dec 19, 2024

Assam MBBS Application Form 2024: Round 2 Registration (Started), Fees, How to Fill

Dec 19, 2024

AMU MBBS Counselling 2025: Dates, Registration, Choice Filling, Seat Allotment

Dec 19, 2024

Minimum Marks Required in NEET 2025 for MBBS Category-Wise

Dec 19, 2024

Quick Facts
Practice Questions
Concept Covered
Concept Video

Begin a career in Medical and Allied Sciences. Admissions Open for

NEET College Predictor

Know possible Govt/Private MBBS/BDS Colleges based on your NEET rank

NEET Companion

Register for Careers360 NEET Counseling & Admission Guidance Service.

TOEFL ® Registrations 2024

Accepted by more than 11,000 universities in over 150 countries worldwide

Manav Rachna-MRIIRS Allied Health Sciences Admissions 2025

Admissions open for Bachelor of Physiotherapy, B.Sc Nutrition & Dietetics ,B.Sc Food Science & Technology

View All Application Forms

News and Notifications

NEET syllabus 2025 out; NMC uploads subject-wise topics

December 17, 2024 - 04:44 PM IST

What changes in NEET UG? Experts’ panel suggests multi-stage exam, security overhaul, simpler process to NTA

December 17, 2024 - 04:22 PM IST

NEET 2025 registration date soon; MBBS, BDS cut-offs in Rajasthan colleges

December 15, 2024 - 06:30 PM IST

Max Healthcare Foundation launches Max Medical Scholarship programme for 100 MBBS Students

December 11, 2024 - 02:51 PM IST

NEET UG 2025 exam date soon; know cut-off for MBBS in top government medical colleges

December 04, 2024 - 08:06 PM IST

MP AYUSH NEET UG 2024 stray vacancy choice-filling ends today; reporting from December 6

December 03, 2024 - 10:26 PM IST

Maharashtra NEET UG Counselling: HC asks medical college to admit student denied MBBS seat in fee row

December 03, 2024 - 06:47 PM IST

Explore on Careers360

NEET

NEET Application Form

NEET Admit card

NEET Syllabus

NEET Answer Key

NEET Cutoff

NEET Result

NEET Exam Dates

NEET Counselling

RPVT

RPVT Application Form

RPVT Admit Card

RPVT Answer Key

RPVT Result

RPVT Seats

RPVT Syllabus

AIIMS Nursing

AIIMS Nursing Application Form

AIIMS Nursing Admit Card

AIIMS Nursing Result

Indian Army BSc Nursing

Indian Army BSc Nursing Application Form

Indian Army BSc Nursing Admit Card

Indian Army BSc Nursing Model Question Paper

Indian Army BSc Nursing Result

By Degree

Top M.B.B.S. Colleges in India

Top B.Sc. Colleges in India

Top B.D.S. Colleges in India

By Branch

Top Pathology Colleges in India

Top Gynaecology and Obstetrics Colleges in India

Top General Medicine and Surgery Colleges in India

Top General Nursing and Midwifery Colleges in India

By Exam

Medical Colleges in India Accepting NEET

By City

Top Medical Colleges in Bangalore

Top Medical Colleges in Chennai

Top Medical Colleges in Kolkata

Top Medical Colleges in Pune

Top Medical Colleges in Mumbai

Top Medical Colleges in New Delhi

Top Medical Colleges in Hyderabad

Top Medical Colleges in Ahmedabad

By State

Top Medical Colleges in Maharashtra

Top Medical Colleges in Karnataka

Top Medical Colleges in Tamilnadu

Top Medical Colleges in Punjab

Top Medical Colleges in Uttar Pradesh

Top Medical Colleges in Rajasthan

Top Medical Colleges in Madhya Pradesh

Top Medical Colleges in Gujarat

Top Medical Colleges in West Bengal

Download Careers360 App

All this at the convenience of your phone

Regular Exam Updates
Best College Recommendations
College & Rank predictors
Detailed Books and Sample Papers
Question and Answers

Scan and download the app

OR

About
Hiring
Magazine
News
Contact
Blogs

Medical Colleges in India
Private Medical Colleges in India
Government Medical Colleges in India
Government Medical Colleges in Uttar Pradesh
Medical Colleges in Tamil Nadu
Medical Colleges in Maharashtra
Medical Colleges in Karnataka
Medical Colleges in Kerala
Medical Colleges in Bihar
Medical Colleges in West Bengal
Medical Colleges in Haryana
AIIMS Delhi
CMC Vellore

NEET 2025
NEET MDS Exam
NEET PG 2024
FMGE 2024
INI CET 2025 January Session
AIAPGET 2024
NEET SS 2024
DNB PDCET 2024
AIIMS Bsc Nursing Exam
AIAPGET 2024

NEET Rank Predictor
NEET PG Rank Predictor
NEET College Predictor
NEET PG College Predictor
DNB CET College Predictor
DNB PDCET College Predictor
NEET MDS College Predictor
Medical E-books
Medical Sample Papers
NEET E-Books
Download NEET Sample Papers

NEET Exam
NEET Counselling 2024
NEET PG Counselling 2024
NEET Cut off
NEET Study Material 2025
NEET Online Preparation
Popular Medical Branches
QnA - Get answers to your doubts
Download App
Counselling Webinars
Online Certification Courses
NEET Question Papers
NEET 2025 Exam Date

Sitemap
Disclaimer
Comment Policy
Privacy Policy
Grievance Redressal

Copyright © 2024 Pathfinder Publishing Pvt Ltd.

National Eligibility cum Entrance Test

NEET 2025 Syllabus

Back to top