Important Physics Formulas For NEET 2025 Exam- Topic-wise Formulas

Important Physics Formulas for NEET 2025: Preparation for the NEET 2025 exam demands thorough knowledge of Physics, which is generally regarded as a difficult but scoring subject. A clear knowledge of Physics formulas is important for solving questions fast. To help NEET candidates, a topic-wise Physics NEET Formula Sheet 2025 has been prepared. It covers important formulas of most asked topics including Mechanics, Thermodynamics, Electrodynamics, Waves, and Modern Physics. Such formulas prove very helpful for instant revision and last-minute study.

NEET 2025: Mock Test (Free) | Biology: Mind Maps & Important Diagrams

NEET 2025: Syllabus | Most Scoring concepts | NEET PYQ's (2015-24)

New: Meet Careers360 experts in your city and get guidance on shortlisting colleges

New Programme: B.E Environmental & Sustainability @ BITS Pilani for PCB Candidates

This Story also Contains

1. Physics Formula Sheet for NEET PDF - Topic-wise and Chapterwise
2. Series LCR Circuit
3. Equations Of Motions Of SHM
4. Electric Potential Due To Continuous Charge Distribution
5. Resistance and Resistivity
6. Parallel Grouping of Resistance
7. Kirchoff's second law
8. Nature of Electromagnetic Waves
9. Total Internal Reflection
10. Young's Double Slit Experiment
11. De-Broglie Wavelength Of An Electron
12. Logic Gates

The NEET UG 2025 exam date is 4th May 2025. Revision gets easier if there is a good understanding of the Physics formulas. Formula-based questions tend to be straightforward and provide an easy chance to get marks if the concept is understood properly. The NEET Physics Formula Sheet PDF includes formulas from the most relevant and regularly asked concepts from the syllabus. It helps in better accuracy, speed, and problem-solving skills which are important components of success in the NEET exam.

Also, check:

• Important Chemistry Formulas for NEET 2025
• NEET Formula Sheet
• NEET Previous Year Question Paper

Physics Formula Sheet for NEET PDF - Topic-wise and Chapterwise

Here are some physics formulas to help you with the revision:

Series LCR Circuit

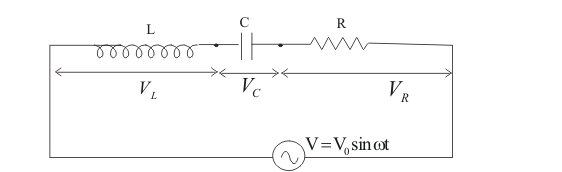
Let 'i' be the amount of current in the circuit at any time and VL, VC and VR be the potential drop across L, C, and R, respectively. Then,
vR=iR→ Voltage is in phase with i
vL=iωL→ voltage is leading i by 90∘
vc=i/ωc→ voltage is lagging behind i by 90∘

using all these, we can draw a phasor diagram as shown below -

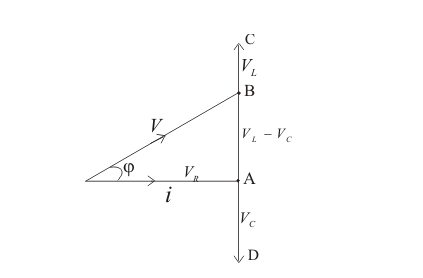
So, from the above phasor diagram, V will represent the resultant of vectors VR and (VL -VC). So the equation becomes -

V=VR2+(VL−VC)2=iR2+(XL−XC)2=iR2+(ωL−1ωC)2=iZ where, Z=R2+(ωL−1ωC)2

Here, Z is called the impedance of this circuit.

Now, come to the phase angle. The phase angle for this case is given as -

tan⁡φ=VL−VCVR=XL−XCR=ωL−1ωCR

Equations Of Motions Of SHM

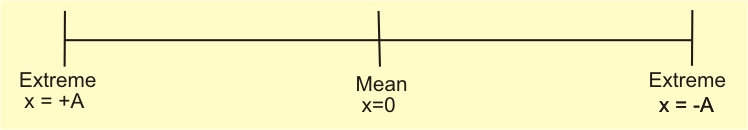
As we know, a=−ω2x

• General equation of SHM

1. For Displacement:-

x=ASin(wt+ϕ); where is initial phase and (ωt+ϕ) is called as phase.

Various displacement equations:-

(1) x= ASinwt ⇒ when particle starts from mean position towards right.
(2) x=− ASinwt ⇒ when particle starts from mean position towards left.
(3) x=ACosωt⇒ when particle starts from extreme position towards
(4) x=− ACoswt ⇒ when particle starts from left extreme position towards Right.

2. For Velocity (v):-

x=ASin(ωt+ϕ)⇒v=dxdt=AωCos(ωt+ϕ)=AωSin(ωt+ϕ+π2)

3. For Acceleration:-

x=ASin(ωt+ϕ)⇒v=dxdt=AωCos(ωt+ϕ)=AωSin(ωt+ϕ+π2)⇒a=dvdt=−Aω2Sin(ωt+ϕ)=Aω2Sin(ωt+ϕ+π)=−ω2x

So, here we can see that the phase difference between x and v is π2
Similarly, the phase difference between v and a is π2
Similarly, the phase difference between a and x is π

• Differential equation of SHM:-

dvdt=−ω2x⇒ddt(dxdt)=−ω2x⇒d2xdt2+ω2x=0

If the motion of any particle satisfies this equation, then that particle will do SHM.

Also read:

• How to prepare for NEET in 30 days?
• How to complete NEET syllabus on time?
• Preparation tips to crack NEET

Electric Potential Due To Continuous Charge Distribution

Electric Potential due to Hollow Conducting, Hollow Non- Conducting, and Solid Conducting Sphere-

In the case of Hollow conducting, Hollow non-conducting, and solid conducting spheres, charges always reside on the surface of the sphere.

If the charge on a conducting sphere of radius R is Q. We want to find V at point P at a distance r from the centre of the sphere.

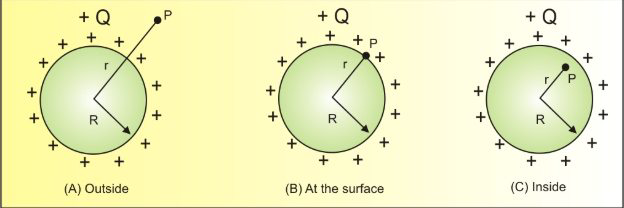
• Outside the sphere (P lies outside the sphere. I.e r>R)

Eout =14πϵ0Qr2=σR2ϵ0r2V(r)=−∫r=∞r=rE→.dr→=14πε0Q→r

- Inside the sphere (P lies inside the sphere. I.e r<R )

Ein =0

Vin = constant and it is given as

V(r)=−∫r=∞r=rE→⋅dr→=−∫∞REr(dr)−∫RrEr(dr)=14πε0∗qR+0⇒V(r)=14πε0∗qR

• At the surface of the Sphere (I.e at r=R)

Es=14πϵ0QR2=σϵ0Vs=14πϵ0QR=σRϵ0

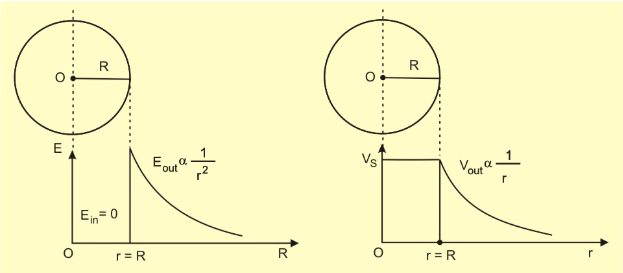
• The graph between (E vs r) and (V vs r) is given below

Electric Potential due to Uniformly Charged Non-conducting Sphere-

Suppose charge Q is uniformly distributed in the volume of a non-conducting sphere of Radius R.

And we want to find V at point P at a distance r from the centre of the sphere.

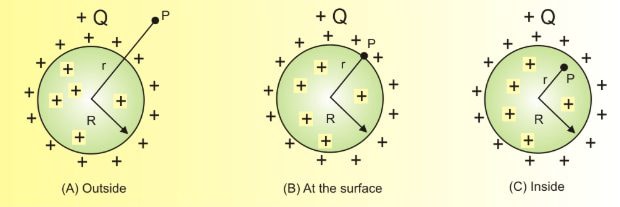
• Outside the sphere (P lies outside the sphere. I.e r>R)

Eout =14πϵ0Qr2Vout =14πϵ0QrEout =ρR33ϵ0r2Vout =ρR33ϵ0r

- Inside the sphere (P lies inside the sphere. I.e r<R )

Ein=14πϵ0QrR3Vin=Q4πϵ0∗3R2−r22R3Ein =ρr3ϵ0Vin=ρ(3R2−r2)6ϵ0

• At the surface of the Sphere (I.e at r=R)

Es=14πϵ0QR2Vs=14πϵ0QREs=ρR3ϵ0Vs=ρR23ϵ0

Note - If P lies at the centre of the uniformly charged non-conducting sphere (I.e at r = 0),

Vcentre =32×14πϵ0QR=32Vs i.e Vc>Vs

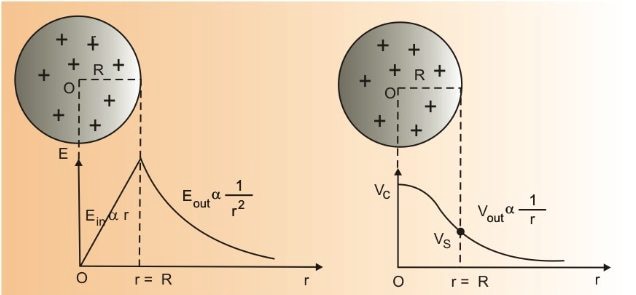
• The graph between (E vs r) and (V vs r) is given below

Resistance and Resistivity

Resistance

For a conductor of resistivity ρ having a length of a conductor=1
and Area of a crosssection of conductor =A
Then, the resistance of a conductor is given as

R=ρlA

Where ρ→ Resistivity
And for a conductor, if n= No. of free electrons per unit volume in the conductor, τ= relaxation time and the resistance of the conductor
Then ρ=mne2τ
for different conductors, n is different
And ρ depends on the n
So R is also different.
Resistivity or Specific Resistance (ρ)

• As

R=ρlA

|f|=1 m and A=1 m2
Then R=ρ

Resistivity is numerically equal to the resistance of a substance having a unit area of cross-section and unit length.

Parallel Grouping of Resistance

Potential is the Same across each resistor, and current is different

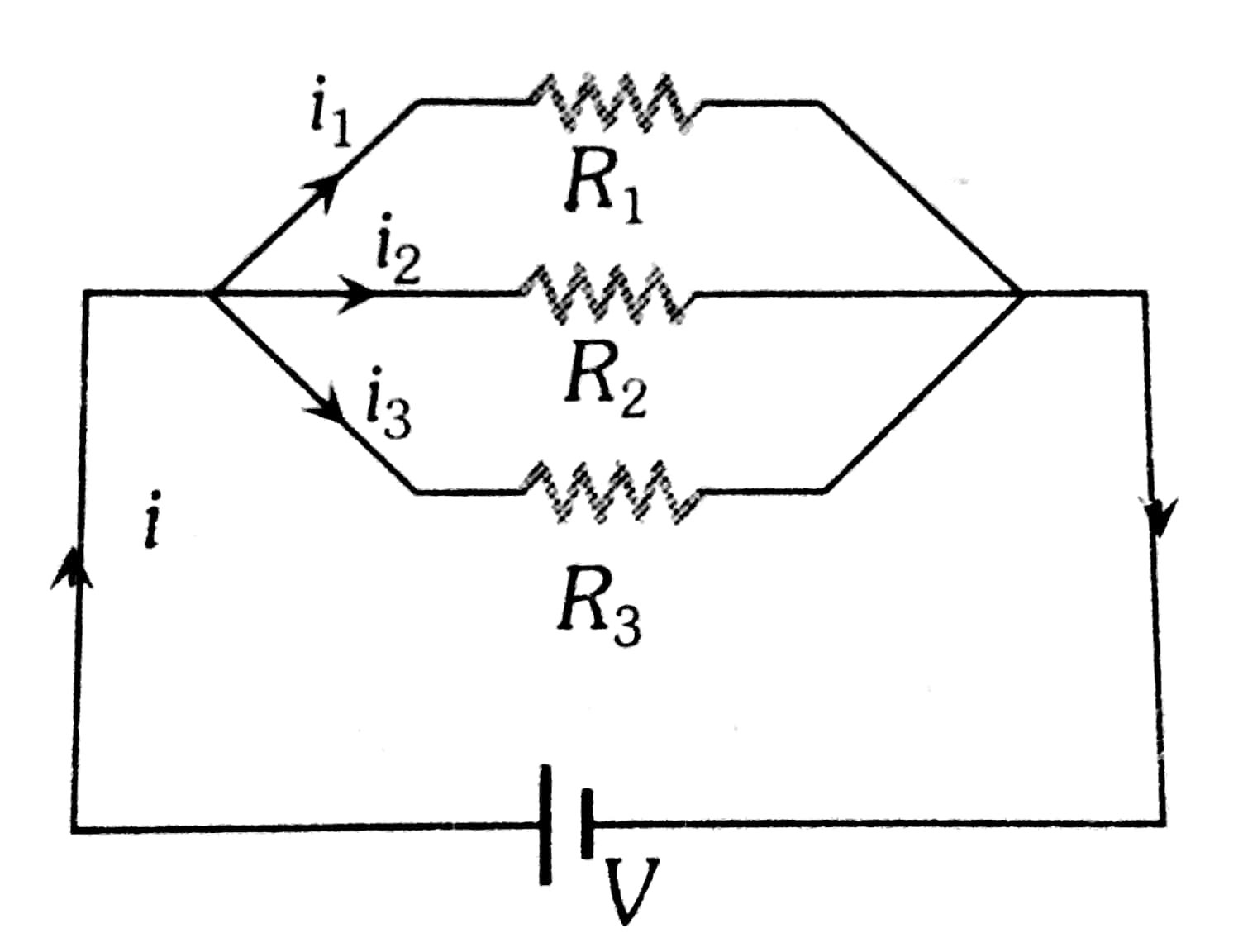
1Req=1R1+1R2+⋯+1Rn

If two resistances are in Parallel:

Req=R1R2R1+R2

• Current through any resistance:

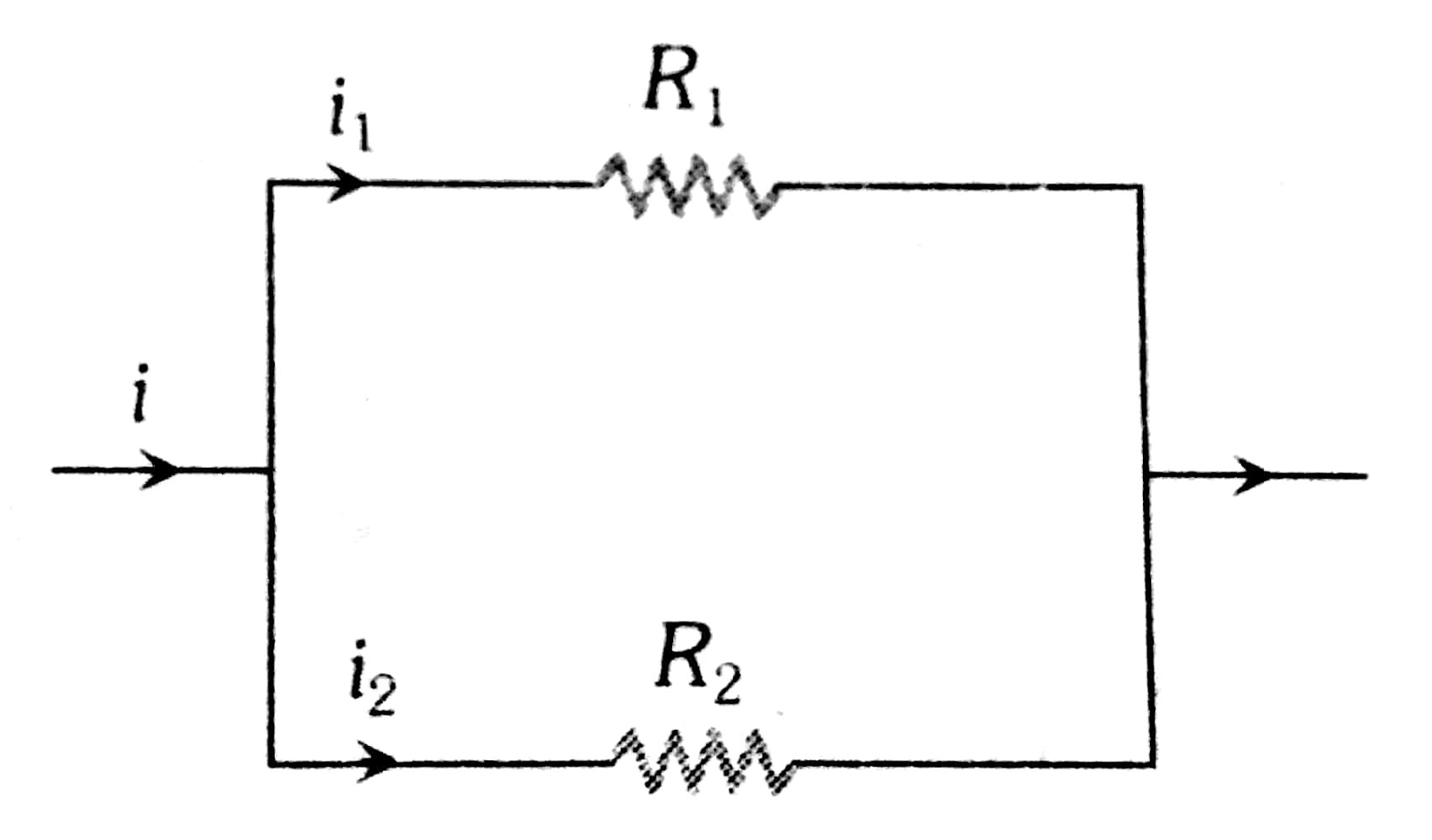
i′=i( Resistance of opposite Branch total Resistance )

The required current of the first branch

i1=i(R2R2+R2)

The required current of the second branch

i2=i(R1R1+R2)

Kirchoff's second law

The algebraic sum of all the potential across a closed loop is zero. This law is also known as Kirchhoff's Voltage law (KVL)

In closed-loop

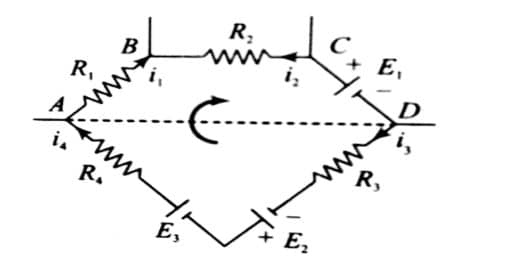
−i1R1+i2R2−E1−i3R3+E2+E3−i4R4=0

Nature of Electromagnetic Waves

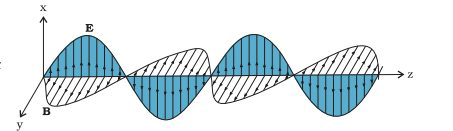
From Maxwell’s equations, we can observe that electric and magnetic fields in an electromagnetic wave are perpendicular to each other and the direction of propagation. Also, from our discussion of the displacement current, in that capacitor, the electric field inside the plates is directed perpendicular to the plates.

The figure given below shows a typical example of a plane electromagnetic wave propagating along the z direction (the fields are shown as a function of the z coordinate at a given time t). The electric field Ex is along the x-axis and varies sinusoidally with z at a given time. The magnetic field By is along the y-axis and again varies sinusoidally with z. The electric and magnetic fields Ex and By are perpendicular to each other and the direction z of propagation.

Now from the Lorentz equation -

F→=q(E→+v→×B→)Ez=Ez0sin⁡(ωt−ky)

Bx=Bx0sin⁡(ωt−ky), where ωk=1μ0ε0

since, ω=2πf, where f is the frequency and k=2πλ, where λ is the wavelength.
Therefore, ωk=2πf2π/λ=fλ
But fλ gives the velocity of the wave. So, fλ=c=ωk. So we can write -

c=ωk=1μ0ε0

It is also seen from Maxwell’s equations that the magnitude of the electric and magnetic fields in an electromagnetic wave are related as -

B0=Eoc

In a material medium of permittivity ε and magnetic permeability μ, the velocity of light becomes,

v=1με

Total Internal Reflection

When a ray of light goes from a denser to a rarer medium, it bends away from the normal, and as the angle of incidence in a denser medium increases, the angle of refraction in a rarer medium also increases and at a certain angle, the angle of refraction becomes 90 degree this angle of incidence is called critical angle (C).

When the Angle of incidence exceeds the critical angle then light ray comes back into the same medium after reflection from the interface. This phenomenon is called Total internal reflection (TIR).

Using Snell's law :

μ2sin⁡C=μ1sin⁡r⟹μ2sin⁡C=μ1 since, sin⁡r=1.⟹sin⁡C=μ1μ2= R.I of rarer medium R.I of denser medium or μ=1sin⁡Cwhen μ1=1 for air and μ2=μ.

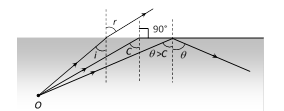
Conditions for TIR :

(i) The ray must travel from a denser medium to a rarer medium.

(ii) The angle of incidence 'i' must be greater than the critical angle 'C' i.e i>C.

Young's Double Slit Experiment

Let d be the distance between two coherent sources A and B with wavelength λ. A screen XY is placed parallel to an opaque screen at a distance D. O is a point on the screen equidistant from A and B. P is a point at a distance x from O

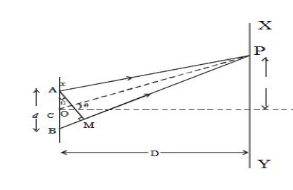
From the above figure, we can see that the waves from A and B meet at P. It may be in phase or out of phase, depending upon the path difference between the two waves,

So, the path difference is =xdD

Assumptions in this experiment -

1. D> d: Since D > > d, the two light rays are assumed to be parallel, then the path difference,

2. d/λ >> 1: Often, d is a fraction of a millimetre and λ is a fraction of a micrometre for visible light.

For Bright Fringes -

By the principle of interference, the condition for constructive interference is the path difference = nλ

xdD=nλ

Here, n=0,1,2…… indicates the order of bright fringes
So, x=(nλDd)
This equation gives the distance of the nth bright fringe from the point O.

For Dark fringes -

By the principle of interference, the condition for destructive interference is the path difference =(2n−1)λ2.here, n=1,2,3… indicates the order of the dark fringes.

So,

x=(2n−1)λD2d

The above equation gives the distance of the nth dark fringe from the point O.

So, we can say that the alternately dark and bright fringe will be obtained on either side of the central bright fringe.

Band Width (β) -

The distance between any two consecutive bright or dark bands is called bandwidth.

Take the consecutive dark or bright fringe -

xn+1−xn=(n+1)λDd−(n)λDdxn+1−xn=λDd

β=λDd

Angular fringe width -

θ=βD=λD/dD=λd

De-Broglie Wavelength Of An Electron

De Broglie’s Equation is given as λ=hp=hmv=h2mK

So for an electron has velocity v attained by it when it is accelerated through a potential difference of V.

then (Kinetic energy gained by the electron) = (work is done on an electron by the electric field)

i.e. K=WE⇒12mev2=eV

So, the De-Broglie wavelength of an Electron is given as

λe=hmev=h2meK=h2me(eV)

using h=6.626×10−34 J and me=9.1×10−31 kg and e=1.6×10−19C
we get

λe=12.27VA(i.e answer will be in A0= Angstrom )
Similarly, we can find De - Broglie wavelength associated with charged particle
De - Broglie wavelength with charged particle-

λ=h2mK=h2mqV
Where K→ kinetic energy of particle
q→ charged particle
V→ potential difference

• De - Broglie wavelength of the proton

using mp=1.67×10−27 kg and qp=e=1.6×10−19C
we get λproton =0.286VA∘
- De - Broglie wavelength of Deuteron
using mD=2×1.67×10−27 kg and qD=e=1.6×10−19C
we get λdeutron =0.202VA∘
- De - Broglie wavelength of an Alpha particle (He2+)
using mα2+=4×1.67×10−27 kg and qα2+=2e=2×1.6×10−19C
we get λα− partical =0.101VA∘

Logic Gates

The five most commonly used logic gates are:

• NOT

• AND

• OR

• NAND

• NOR

NOT Gate -

A NOT gate is also known as an inverter because it simply inverts the input signal. It is a simple gate with one input and one output. So, the output is ‘0’ when the input is ‘1’ and vice-versa.

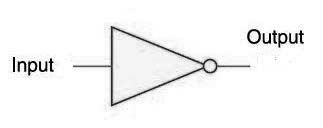
A is input

Y is output

Y=A¯

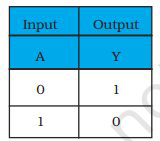
The truth table for a NOT gate is as follows:

AND Gate-

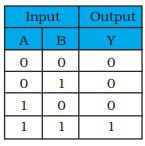
An AND gate has two or more inputs and a single output. In this gate, the output is 1 (high) only when all the inputs are 1 (high). The most commonly used symbol for an AND gate is as follows:

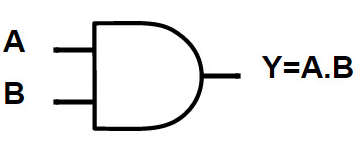
A and B are inputs

Y is output

Y=A.B

The truth table for the AND gate is as follows

OR Gate-

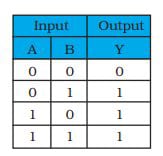
Like AND Gate, OR gate also has two or more inputs and one output. For this Gate, the logic is that the output would be 1 when at least one of the inputs is 1. It means that when the output is high, any of the inputs are high. The commonly used symbol for an OR gate is as follows:

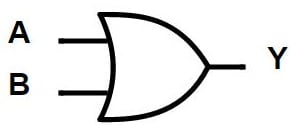
A and B are inputs

Y is output

Relation between input and output

Y=A+B

The truth table for an OR gate is as follows:

NAND Gate-

A NAND gate is an arrangement of AND gate followed by a NOT gate. The output is 1 only when all inputs are NOT 1, or the output is high when at least one is low. These are also called Universal gates. The commonly used symbol for a NAND gate is as follows:

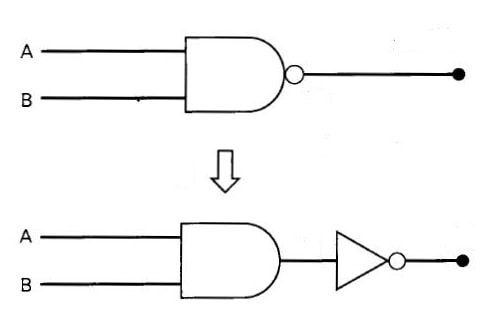
Y=A⋅B―

A and B are inputs

Y is output

NOT + AND gate

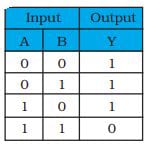
The truth table for a NAND gate is as follows:

NOR Gate-

Like the NAND Gate, the NOR Gate is also an arrangement of an OR Gate followed by a NOT Gate. In this, the output is 1 (High) only when all inputs are 0 (Low). These are also called Universal gates. The commonly used symbol for a NOR gate is as follows:

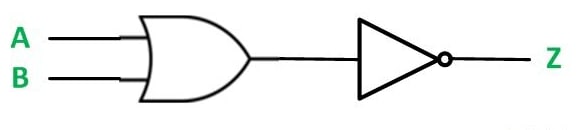
Y=A+B―

A and B are inputs

Y is output

NOT + OR Gate

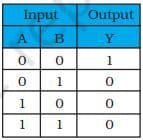
The truth table for a NOR gate is as follows:

Also Read:

• Easiest Section in NEET 2025 Syllabus
• Modern Physics Formula for NEET
• NEET 2025 Sample Paper